The Diverse Mechanical Functions of Claws within Locomotion and Predator-Prey Relation
Liela Andringa, Alan Fu, Alex Jung, Oscar Cruz Hernandez
Abstract
Claws are the ultimate tool in any animal’s survival kit. With an array of purposes for different species across the animal kingdom, the claw has many interpretations that can be analyzed for a deeper understanding of its power. This research paper analyzes the diverse ways that physics is incorporated into the mannerisms of animal claws. All physics-based aspects of the claw are studied through four different animal classifications: arboreal, reptilian, volant, and marine animals. To begin, climbing of arboreal animals is examined to understand the energy exchange, dynamics, and the limitations of the claw. Next, reptilian animals are investigated to further apprehend the application of mobility and sharpness of reptile claws. Subsequently, the physics of perching within passerines and the mechanical adaptations of claws for prey within raptors is analyzed. Finally, the claws of marine animals are studied to better understand how the physics behind them make them beneficial for hunting and protection. Each animal group in this study reveals applications and case studies of everyday physics.
Introduction
A claw can provide great advantages to different animals in many scenarios. Whether they are used for self-defense, climbing, hunting, or general locomotion, claws are an essential component of an animal’s survival and are a fascinating tool to investigate. Through the analyses of various animals, this paper will delve into the many physics applications and components of different types of claws. As will be demonstrated, there is a consistent pattern whereby every function of the claw is supported by a physics-based explanation that justifies its purpose.
Claw physics in arboreal animals
An immense amount of energy is required to climb from the trunk of a tree to the top of a tree branch; however, arboreal animals can perform this demanding task within seconds, going from point A to point B with ease. This provokes the question: how exactly do arboreal animals take advantage of physics to excel in climbing? The answer behind this curiosity shines light on a specialized component of an arboreal animal’s anatomy: the claw.
Kinetic and static energy involved in climbing
The claw is an immensely powerful tool, which provides great advantages to many arboreal animals. However, it is not immediately evident how it gains its strength and power when this advantageous tool is mainly composed of a relatively soft protein, namely, keratin. What are the special factors that make this tool for multiple different animals so strong and reliable? Well, the short answer has to do with the energy conversions between the claw’s kinetic and static energy.
Taking into consideration Mohs’ scale of hardness, which is presented in Figure 1, keratin scores around 2.5. For such a strong and widely used tool, this is a low score, especially in comparison to many other materials that are found in nature. If this protein is inferior in hardness to many objects in nature, how is it capable of striking and penetrating objects that score a higher value on Mohs’ hardness scale compared to itself, such as the structures which animals use their claws to climb on?

Fig. 1. Mohs Hardness Scale [Adapted from Science Notes, Courtesy of Anne Helmenstine, 2022]. In consideration of this research paper, fingernail hardness value is equivalent to the hardness value of keratin in animal claws (since both are made of keratin).
Another factor must come into play to make this logical: energy. Research has suggested that kinetic energy must be considered in the situation where claws strike materials of higher hardness values (Rothschild et al., 2013). In this study, it was noted that tigers were capable of using their claws to damage bone with a hardness value of around 5 – twice that of the keratin of claws (Rothschild et al., 2013). This demonstrates the importance that kinetic energy has on arboreal animals’ ability to climb on specific materials. Applying this concept to a specific example, squirrels are capable of climbing on many different surfaces with a larger hardness value than keratin, including brick, which scores a hardness of around 5 to 7 on Mohs scale. This means that the kinetic energy from the claws gripping the surface is a key component for all animals that need to climb to survive. This way, animals can not only climb trees, but also elements with larger hardness values such as concrete (6-7 hardness value), steel poles (4.5 hardness value), and large rocks (1-10 hardness value) (Jenkins F. A., 1974). Climbing on a surface with a larger hardness value in comparison to an arboreal animal’s claws can be demonstrated in Figure 2.

Fig. 2. Arboreal animal climbing brick [Adapted from Jenkins F. A., 1974]. A squirrel successfully climbs up a brick wall despite brick scoring a larger hardness value in comparison to keratin.
Application of the claws to physical dynamics
A simple observation of a squirrel climbing up a tree makes it evident that the claw can greatly contribute to their agility; however, it is also the physical dynamics behind the claws that play a significant role within the squirrel’s climbing activity.
For trees with a circumference small enough for the animal to wrap its limbs around the diameter, the squirrel can swiftly climb up with the help of friction from its paw pads; however, for trees with a larger circumference, a squirrel needs more than just friction to move to its next destination (Jenkins F. A., 1974). With the help of the claws, the squirrel can anchor its limbs onto the surface of the tree without having to wrap its limbs around the trunk to get a firm grip. During this process, the claws help distribute the mass of the squirrel – by taking up a larger surface area on the tree – and assist the squirrel in locating its center of gravity closer to the surface that it is climbing. With its claw’s aid in creating a closer center of gravity, it helps stabilize the squirrel’s balance and is advantageous because the gravitational vector of the mass will be supported by the limbs that are anchored by the claws – which counteracts the gravitational force by creating normal forces where the claw is in contact with its climbing surface (Lammers, A. R., & Zurcher, U., 2011). On the contrary, if the center of gravity is considerably far from the surface on which it is climbing, there will not be enough normal force acting on the claws and limbs for the animal to hold its own mass against the gravitational force acting on the animal. Fortunately, with mass distribution refined, this allows the gravitational forces on arboreal animals’ bodies to disperse around their volume instead of having all their weight concentrated in one central spot (Lammers, A. R., & Zurcher, U., 2011). This can be illustrated through Figure 3.
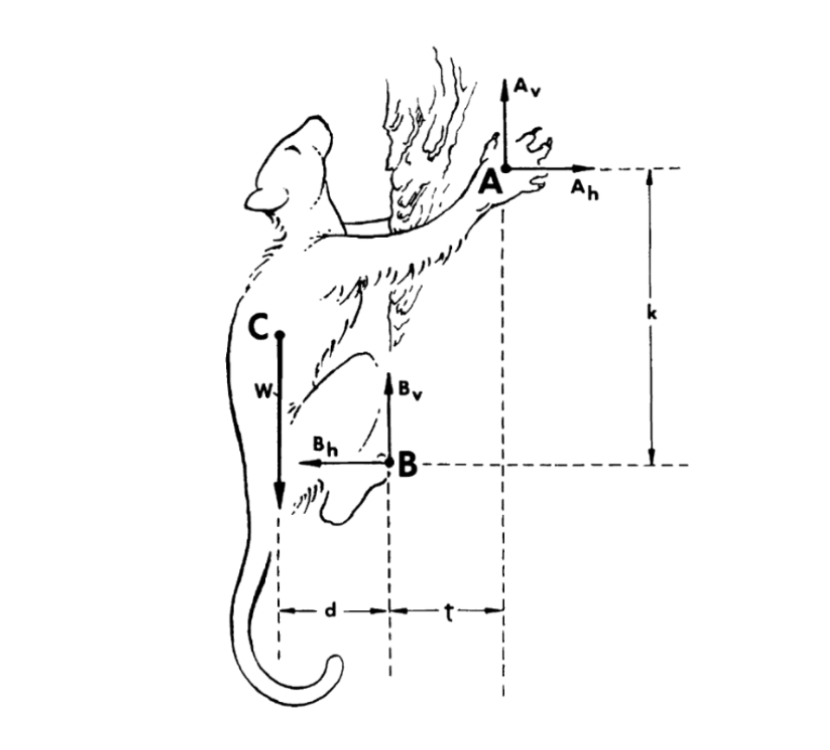
Fig. 3. Free Body Diagram of an arboreal animal climbing up a tree [Adapted from Jenkins F. A., 1974]. This shows the gravitational force acting on the animal (force vector C) and the counteracting normal forces (Av and Bv). Forces Ah and Bh act as gripping support against the tree by using forces that cancel each other out.
In addition to weight distribution across the span of the claws, climbing can only be effective if the claw anchors onto the tree with respect to the climbing surface, at an angle of 90 degrees or higher (Jenkins F. A., 1974). This is important because the underside of the claws is what supports the distributed gravitational force, and if the angle of the grip is less than 90 degrees the claws will anchor poorly. To illustrate, imagine a weak claw grip (a claw grip with less than 90 degrees with respect to the climbing surface) as a ramp (as shown in Figure 4). The horizontal component of the normal force can potentially cause the claw to slide off the anchoring point if the claw is not 90 degrees or higher between the tree’s surface and the underside of the animal’s claw (Jenkins F. A., 1974). Whereas, if the angle is equal to or more than 90 degrees, the normal force created by the claw grip could support the animal’s weight.

Fig. 4 How angles influence the support of a claw grip [Courtesy of Jung, 2022]. This diagram shows that if the claw grip angle is 90 degrees, the gravitational force is completely supported by the claw’s normal force.
Physical limits of the claw
After looking into all the amazing abilities that are provided within this useful tool for arboreal animals, it is justified to interrogate whether there is a limitation to its many wonders. Unfortunately, there is a limitation to the claw’s ability when considering the mass of the animal in proportion to the amount of force that the claw can withstand.
Claw strength is dependent on the width and radius of the base of the claw. This makes sense since the stress on the tip of an animal’s claw creates a large moment of inertia that has an accelerated increase from when there is pressure (caused by the force of the mass) on the tip of the claw. (Labonte, D., & Federle, W., 2015). This increases the probability of a fracture and can lead to a buildup of stress in the claw. However, stress decreases closer to the claw base because the moment of inertia also decreases closer to the base of the claw. Inertia decreases naturally as the radius of rotation decreases; therefore, inertia decreases closer to the claw bed (known as the “rotational axis”) which creates less stress on the claw (Engineering ToolBox, 2005). Knowing this, the limit of arboreal animals’ claws depends on the animal’s mass which contributes to the force applied to the tip of the claw. Therefore, stress of the claw is proportional to the mass divided by the radius of the claw tip – which can be found through analysis of the logarithmic growth spiral of the claw. Moreover, the logarithmic growth spiral creates the shape of the claw and generates strength within the system as it is dependent on a power law between the length of the claw and its radius. This shape is called the power cone (Evans et al., 2021). Overall, the analysis of the logarithmic growth of the claw provides a reliable radius to be used to find the stress within the claw, using Equation 1.

Eq. 1 Relationship between mass and the limitations of the claw [Labonte, D., & Federle, W., 2015].
In Equation 1, stress is represented by σ. Mass is represented by m. Radius of the claw tip is represented by R. It is evident that stress is proportional to the mass of the animal divided by the radius of the claw tip squared.
Claw physics in reptilian animals
Reptiles are land animals that mostly lay eggs and have claws (Zug, 2022). Even limbless snakes have spurs, which have a similar build to claws in terms of their keratinized construction (Palci, 2020). Reptiles differ from amphibians who do not have claws and spend most of their life close to or in water. Being on land, reptiles use their claws to move, climb, dig, and hold prey (Alibardi, 2020).
Adaptation of claws for mobility
Claws are used by reptiles mainly to climb, sometimes alongside adhesive pads which are found in lizards (Russell, 1988). The claws offer increased traction and allow the animal to climb more types of surfaces under various conditions. To this end, two phenomena can be observed.
The first phenomenon is claws oriented in opposing directions, similar to birds. The reptiles that bear this characteristic are the chameleons; a specific example would be the Ch. calyptratus (Spinner 2014). They have adhesive pads that are complemented by claws pointing in the same direction once the digits are wrapped around branches. These claws then apply pressure on a perpendicular branch. For smaller branches, the pes and the adhesive pads are enough to grip the branch as the pes, the foot, surrounds the branch as seen in Figure 5. The claws are used to find asperities or indent the branch to stay firmly on it. This allows the animal to get hold of much larger branches than the pes alone would be able to. The extreme case seen in Figure 5 is when the combination of the claw and digits wrap around 40% of the branch and still maintain a grip at an inclination of 90°.

Fig. 5. The capability of chameleons to grasp a branch based on diameter [Adapted from Spinner 2014]. The white line in the graph is a male chameleon and the black and gray lines represent two female chameleons.
The second type of claw configuration is when the digits are in the same direction, however, the claws are in different directions. An example of a reptile that bears this characteristic is the A. seychellensis (Russell, 1988). As seen in Figure 6, the claws are pointing in two directions due to how the adhesive pads are positioned on the digit. In the case of this lizard, there are two claws pointing laterally left, and 3 right for the right hind pes, mirrored by the left one. The pads take up a lot of space and push the claws into the asymmetrical position needed for an ideal grip (Russell, 1988). One would think that this is a disadvantage, but instead it helps to grip branches. In Figure 6, we can see how the digits grab the branch in a diagonal way. The claws end up being parallel to each other once the branch is grabbed and the reptile grips the branch if it is large enough, similar to the chameleon.

Fig. 6. Ventral view of the A. seychellensis and the hind pes of an iguana [Adapted from Russell, 1988]. The animal to the left is an iguana and the animal to the right is a gecko on a very large branch.
As a singular claw could never hold the whole weight of a large animal, reptiles use the mobility of their digits to reposition the claws in the same direction while climbing to redistribute the force between the multiple claws (Labonte 2015). This is usually used among smaller animals such as lizards and insects, but it ultimately works for larger animals as well when they are not too heavy, to avoid breaking their claws in the process. Considering that some animals have 5 digits per paw, this would mean that the entire weight would be distributed over 20 claws. This may be seen in the behavior of the Komodo dragon. It has long claws that grant it enough traction to support and lift its weight of approximately 25 kg (Komodo dragon, 2019). This ability allows the younger and lighter Komodo dragon to escape older adults that may have cannibalistic tendencies in a time of food scarcity (Poma, 2010). This is because a fully grown Komodo dragon gets so heavy that it is no longer able to sustain its own weight with the claws even with the distribution of weight force. The claws of an adult Komodo dragon are duller, which helps support the weight of the animal on the ground. When a claw falls off from a reptile, depending on where it fractures, it sometimes never grows back, highlighting the importance for the reptile in question to develop sturdy claws that are also functional.
There is also a type of ground dwelling lizard that moves with its belly sagging on the ground. This lizard, the T. scincoides, seen in Figure 7, has no adhesive pads, therefore it must rely on claws and its own skin for traction (Stefan, 2014).

Figure. 7 Image C illustrates the marks of the winding movement of T. scincoides [Adapted from Stefan, 2014].
The T. scincoides‘ belly is so big that it becomes an obstacle and the legs are forced to move around. This makes the lizard walk in a zigzag that may seem like an inconvenience but is helpful when climbing uphill. It is just like when one rides a bike up a steep hill: one finds oneself going in a zigzag as shown in Figure 8 to not fall and travel up while using a reasonable amount of power (MacDonald, 2013).

Fig. 8. The difference between different ascending path angles (Courtesy of Cruz, 2022). The path in orange is the path that a T. scincoides would take. More inclined path (a) is longer but has a lesser angle compared to (b).
It is true that one can reduce the gear of a bicycle to make it easier, but the animal’s body does not have built in gears. The T. scincoides is prevented from walking straight due to its different form but that serves a function as it can use the diagonal direction of its claws as traction to advance in slippery surfaces such as clay, showing the complementarity of form and function, as seen in Figure 7.
The sharpness of claws in reptiles
Reptile claws are slightly sharpened during development stages but are also passively sharpened by natural factors. Claws consist of two elements, the unguis, the upper part, and the sub unguis, the lower part. As the claw develops, the sub unguis grows in a slower manner that forces the unguis to wrap around and condense at the tip, giving it a pointy shape (Alibardi, 2020). This continues at a reduced pace during adulthood. After extensive use on harsh surfaces, these claws are sharpened, as seen in Figure 9. This is due to the sub unguis not being as strong as the unguis. The desquamation, or flaking, of the sub unguis maintains the conical shape of the claw (Alibardi, 2020), meaning that it stays pointy and sharp.

Fig. 9. The desquamation of a claw and the pressure of a claw against skin (Courtesy of Cruz, 2022). The dotted lines represent the part of the claw (a) that has been filed down due to everyday activity. Image (b) represents the pressure against the skin of a Komodo dragon’s prey.
The claws of reptiles never become razor blades as one might think. The profile of claws may be, among others, a sturdier horseshoe shaped (Alibardi, 2020) construction for bigger claws, or an arrow tip for lizards (Russell, 1988). This does not mean that the sheer force of the animal is not enough to dig into its prey and then scratch or injure the prey. For example, the Komodo dragon, which can weigh up to 136 kg (Poma, 2010), can penetrate and hold down its prey with duller claw tips due to its larger weight. We must remember that the formula for pressure includes force and area.

Eq. 2 Equation for pressure.
This means that if the reptile pounces on a prey, the area of its 10 dull claws of 0.004 meter would be:

This increases the pressure on any part of the skin penetrating it and subsequently tearing it if the predator swipes the prey.

For the claw on the left to puncture the skin like in Figure 8, the required pressure is 689 kPa (Rhett, 2021). In the context of the Komodo dragon (Poma, 2010), the prey is bitten and released, letting it slowly die due to shock.
Claw physics in flying animals
Passerine claw perching forces and surface influences
More than half of all bird species fall under the order of Passeriformes, an order distinguished by a claw arrangement of three claws forward and one backward that enables perching. The prevalence of perching behavior within birds is not without cause, as the advantages it creates are numerous. A major benefit is that resting or feeding on perches helps passerines, like the male painted bunting shown in Figure 10, avoid predators. Perching also grants birds excellent vision of their surroundings and enables their songs and calls to be heard across a wider range.

Fig. 10. Male painted bunting perching [Adapted from Macaulay Library, Courtesy of David Hollie, 2014].
The angulation of the perching bird’s claws is intermediate amongst birds, being more angled than the flatter claws of ground-dwelling birds and less angled than the more hooked claws of climbing birds (Feduccia, 1993). This grants them a more generalist claw shape, suited to the varied nature and activities of passerines (Pike & Maitland, 1999).
When engaging in perching, birds will initially slow down using their wings and stretch out their claws to prepare for the landing impact. Remarkably, if any claws slip off an asperity of the perch, birds will make claw movements as fast as 1-2 ms using energy stored in a tendon, and the low inertia of the claw, to stabilize onto the perch (Roderick et al., 2019). Furthermore, the use of load sharing over multiple claws allows birds to reduce the force applied to each individual claw (Jiang et al., 2018). Another method birds use to stabilize on their perch is to drag their claws across the surface to seek better asperities and increase friction force, especially if the surface is more difficult to grasp due to its large diameter or low friction (Roderick et al., 2019). Indeed, we can see in Figure 11 that the expected value of maximum tangential force (FT) over normal force (FN) increases as drag distance increases, especially for surfaces with larger asperities such as Sweet Olive (SO), Coast Live Oak (CLO), and birch.

Fig. 11. Expected value of maximum tangential force over normal force against drag distance on Sweet Olive (SO), Coast Live Oak (CLO), foam, birch, Floss Silk (FS), and Teflon [Adapted from Roderick et al., 2019].
This dragging behavior enables birds to consistently land on a diverse number of surfaces, allowing them to access a wide variety of perches. Of course, birds do not only rely on asperities for stable perching: they use friction forces generated by their toe pads and claws against the surface to counteract forces pushing them off the perch as well. While toe pads, with their soft nature and greater contact area, allow for consistent frictional forces, claws enable frictional forces created by surface deformation and fit onto local surface irregularities. Surface deformation is especially important for soft surfaces, as although depth penetration may only be a small fraction of the claw’s outer arc length, it can be a major factor in maintaining a stable grip (Roderick et al., 2019). A diagram of the forces acting upon the bird’s body may be seen in Figure 12.

Fig. 12. Representation of forces on a perching bird’s body, where forces Fy and Fx as well as torque T must be matched by friction forces from the claws and toe pads [Adapted from Roderick et al., 2019].
Friction ratios of tangential force over normal force from the claws can be over eight times those of the toe pads, and while the friction forces supplied by claws are less consistent, it is clear that they are extremely helpful in counteracting forces on the bird’s body through friction with the surface (Roderick et al., 2019). Graphs comparing the ratio of tangential force to normal force against time for both toe pads and claws can be found in Figure 13.

Fig. 13. Graphs of tangential force over normal force against time for toe pads (above) and claws (below) for a representative test [Adapted from Roderick et al., 2019].
As we can see, claws can reach a much greater Fmax than toe pads. It was also found that increasing claw angle would increase generated friction forces on rougher surfaces, although this would not take effect on smooth surfaces, perhaps explaining the passerine’s mean claw angle: while it is more curved than the claws of ground-dwelling birds, it is not overly so, allowing it to grip on rough surfaces while still enabling other tasks such as foraging and food manipulation (Roderick et al., 2019).
Raptor claw adaptations for hunting
While perching certainly affords many great advantages to birds who make use of it, the functions of claws certainly aren’t limited to locomotion. Raptors, also known as birds of prey, use them as one of their primary tools to capture and kill prey. They are admired for their exceptional abilities that serve this purpose, from extremely high speeds to sharp eyesight. However, the methods with which different orders of raptors use their claws in their hunt differ, which manifests itself in the physics behind their claw usage as well as the way their claws have evolved. We will be examining Accipitridae, Falconidae, and Strigiformes, of which an example is shown from each family in Figure 14, and compare the mechanics of their claws.

Fig. 14. Images of Strigiformes, Accipitridae, and Falconidae [Adapted from Audubon, Courtesy of (A) Robert Holden, (B) Bonnie Block, and (C) Ann Pacheco]. (A) displays a snowy owl, Strigiformes, (B) displays a bald eagle, Accipitridae, and (C) displays a peregrine falcon, Falconidae.
Accipitridae use their claws for hunting in the most conventional way, using them to stab into the prey’s body and constrict the prey to death through asphyxiation or internal organ damage. Falconidae mainly damage prey through the initial strike impact, with finishing blows dealt by the beak; the claws are predominately used to restrain the prey after initial impact. Strigiformes lie on the other side of the spectrum of Accipitridae, using their claws mainly to restrict and kill their prey primarily through their beak. The reason for these differing methods stems from their desired prey: Strigiformes hunt small prey like mice, and thus focus mainly on the capture and restraint of prey, while Accipitridae target large prey as well, like waterfowl, which can be more difficult to kill, leading to their use of claws offensively, while Falconidae lie somewhere in-between (Fowler et al., 2009). These specializations reveal themselves both in the physical shape of claws from each family as well as their grip forces. Let us first examine claw curvatures in Table 1, which are represented as ratios between individual claws to account for differences in bird size and are measured through both the inner and outer curvature. The D-I claw points backwards and DII-IV claws point forward.

Table 1 Representations of mean claw ratios and uncertainties between D-I/D-IV, D-II/D-IV, D-III/D-IV, D-II/D-III for Accipitridae, Falconidae, and Strigiformes [Adapted from Fowler et al., 2019].
As we can see, Accipitridae have by far the largest curvature, with Falconidae in between and Strigiformes with the least curvature on average. This data fits with the previous hypotheses, as Accipitridae require the curvature to pierce into the bodies of their prey, while Strigiformes primarily use their claws to restrain prey, and thus have lower curvature to maximize grip efficiency (Fowler et al., 2009).
Another important physical measure of claw usage is grip force. As Accipitridae primarily kill their prey through their claws, we would expect them to have a stronger grip force compared to Falconidae, who focus on immobilization through initial impact speed and finishing prey with their beak. Indeed, an examination of estimated forces of jaws and beaks shows that they fit well with each family’s specialization, as shown in Table 2.

Table 2 r2 values and P values for estimated forces of jaws and feet for Accipiter and falcons [Adapted from Sustaita, 2007].
Aligning with the hypotheses of Sustaita, Accipitridae have lower estimated forces for their jaws and higher estimated forces for their feet, as compared to Falconidae, which follow the opposite pattern. Although Strigiformes were not examined by this paper, we can make predictions that they would follow the pattern and have higher jaw forces and lower feet forces than Falconidae, given their specialization in killing mainly through their beak.
The many adaptations raptors have made to best hunt their chosen size of prey are both fascinating and logical. Accipitridae require claws to pierce into large prey, having the highest claw curvature and grip force, while Strigiformes, who focus on small prey, have the lowest claw curvature, and Falconidae sit in the middle in claw curvature and below Accipitridae in grip force due to their varied choice of prey. However, birds are not the only creatures with evolved claws targeting their choice of prey; some marine animals also follow this adaptation.
Claw physics in marine animals
Pinniped claw use for prey processing and terrestrial motion
Seals are some of the many marine animals that possess claws. Classified as pinnipeds, there exist quite a few different families within the clade. Various characteristics distinguish the families, including the possession of claws or lack thereof. While all pinnipeds have flippers and are carnivorous, the exact type of food they eat distinguishes which pinnipeds possess claws and which do not. Those with clawed fins require their claws to consume their prey.
Within the pinniped clade, the family of otariids (fur seals and sea lions) possess clawless forelimb flippers while the family of phocines (northern true seals) possess webbed paws with sharp claws, as shown in Figure 15.

Fig. 15. Clawless flippers of Otariid pinnipeds (a & c) compared to the clawed flippers of their Phocine pinniped counterparts (b & d) [Adapted from Hocking et al, 2018].
Otariids typically hunt smaller prey and will swallow them whole, although they occasionally catch larger prey and bring them to land in order to shear them into smaller, swallowable pieces. On the other hand, phocines have the freedom to regularly hunt for larger prey because they are able to use their claws to securely hold their catch during food processing. Phocines will use their claws to grasp their prey while their teeth dig into the sought-after bite. The phocines will then arch their jaw backwards while simultaneously yanking down with their forelimbs in a ‘hold and tear’ method, shown in Figure 16, that enables them to rip large fish into more manageable pieces for swallowing (Hocking et al, 2018). In this method, phocines use their claws to gain a firm enough grasp of their prey so that they can employ strong enough tensile forces to overcome the tensile strength of the fish’s flesh, and ultimately tear it.

Fig. 16. Phocine using its claws to firmly grasp its prey while biting and stretching it to overcome the tensile force of the fish’s flesh to tear it [Marx, F. G., 2018].
The tensile strength of their prey’s flesh ultimately determines the tensile forces required to tear it, as well as the corresponding grip strength required by the phocine claws to garner a firm enough grasp on the fish. Therefore, quantifying the tensile strength of the flesh of phocine prey is imperative in eventually estimating the exact amount of tensile forces and complementary grip forces required by phocines to process fish for consumption. In still-developing tensile strength test methods, an example of which is shown in Figure 17, researchers are honing their testing mechanisms to match the motion and caliber of phocine food processing. The objective is to quantify the force required to tear a standardized block of salmon muscle, and then use this data to determine the tensile strength of the fish’s flesh. Considering that the phocine diet consists considerably of salmon, among other large fish, this data could be used to estimate the magnitude of the forces and grip strength of phocine claws used in processing their prey (Ashton et al, 2010).

Fig. 17. A sample of salmon flesh after a complete tear by a tensile strength test apparatus used to experimentally determine the tensile strength of salmon flesh [Ashton et al, 2010].
Although the possession of claws allows pinnipeds to hunt for larger meals, accommodating the claws prevents the flippers from being as streamlined. This means that those with claws lose agility in the water due to their paws having a lower hydrodynamic efficiency. Thus, it is a tradeoff between diet and marine mobility. However, there is no such tradeoff when it comes to terrestrial mobility. In fact, claws improve their ability to move on certain surfaces, particularly the slippery rock surfaces that seals are often found lounging on (Garrett, J. N., & Fish, F. E., 2015).
Phocines traverse many different types of surfaces, including ice, snow, sand, rocks, and algae. Their movement across each of these different terrain types depends on whether they are sliding or climbing, as well as the angle of incline and coefficient of friction of the surface. In terms of sliding, phocines will glide down surfaces at an angle greater than or equal to 15° so long as there is minimal friction (algae covered surfaces and slippery, wet rocks). In terms of climbing, phocines can reportedly ascend slopes with angles of incline greater than 60° due to their ability to firmly grip the terrain with their claws (Garrett, J. N., & Fish, F. E., 2015).
Pistol shrimp and amphipod claw use for hunting prey and protecting oneself from predators, respectively
Of the marine animals that possess claws, pistol shrimp and amphipods use theirs in one of the most unique ways. Belonging to the Crustacean taxon, it is no surprise that both pistol shrimp and amphipods bear a significant likeness. The most notable similarity between the two crustaceans is their unmistakably large claws, shown in Figure 18. Technically considered pincers because they exist as a limb of a crustacean, one can still categorize these appendages as claws, given that pincers are a type of claw subset, and will therefore analyze the complex physics involved in their functionality.

Fig. 18. Pistol Shrimp (left) [Allen, N., 2020] and Amphipod (right) [Willis, K., n.d.].
While the pistol shrimp, with its protective carapace, uses its formidable claw to hunt prey, the carapace-lacking amphipod uses its powerful claw to protect itself against predators. Although the ultimate purpose of each claw differs between the species, the overall mechanics are the same. Both species’ claws work to weaponize the phenomenon of cavitation.
Cavitation, shown in Figure 19, is the rapid formation and quick collapse of vapor bubbles produced within the confines of a liquid. It occurs when the rapid displacement of a liquid decreases that displaced liquid’s pressure to the point where it falls below the liquid’s vapor pressure. This sudden pressure discrepancy results in the rapid formation of vapor bubbles within the surrounding liquid. The vapor bubbles expand and then quickly collapse due to the overpowering pressure of the surrounding liquid. This collapse of the bubbles releases intense energy in the form of shock waves with enough power to stun a predator or kill prey (Ijiri et al, 2018).

Fig. 19. Artificial process of water jet cavitation mimicking the naturally occurring phenomenon generated by pistol shrimp and amphipods [Ijiri et al, 2018].
Since the mechanism of the claw used to create cavitation is essentially the same for both pistol shrimp and amphipods, this paper will delve into explaining the process of the pistol shrimp’s claw to avoid redundancy and confusion on the topic.
Pistol shrimp possess a dominant claw as well as an auxiliary claw. As the descriptor ‘auxiliary’ implies, the reserve claw will grow to replace the dominant one if an amputation occurs. The large, dominant claw is known as the large chela. The large chela itself consists of the dactylus and the propodus. The dactylus serves as the mobile portion of the claw, able to snap close and firmly fix itself to the adjacent propodus portion of the claw. Protruding from the dactylus is the plunger, which fits sheath-like into the socket of the propodus. This anatomy, shown in Figure 20, lends precisely to the function of the claw (Au, W., & Banks, K., 1998).

Fig. 20. Diagram of the large, dominant claw of pistol shrimp [Au, W., & Banks, K., 1998].
When the pistol shrimp opens its large chela, water fills the socket of the propodus. The pistol shrimp will then rapidly close its claw, forcing the dactylus to snap against the propodus, which drives the plunger into the socket, expelling the water from within the socket through the narrow water jet groove. The ejected jet of water possesses a velocity estimated to be about 25 m/s. This high velocity is expected to produce pressure drops of approximately 300 kPa, which would be enough to vaporize the water and form localized cavitation bubbles. These cavitation bubbles will then collapse, producing incredibly high pressures of approximately 200,000 kPa as a result of suddenly decelerating surrounding liquid (Koukouvinis et al, 2017). This rapid deceleration of the liquid surrounding the collapsing cavitation bubbles occurs because although the initial velocity of the water jet is high, it rapidly diminishes. Research experiments conducted by Herberholz and Schmitz found that a water jet with a velocity of 6.5 m/s resulted in a deceleration to 0.2 m/s within the initial 0.02 seconds. This indicates a significant average acceleration of -315 m/s of the water jets produced by pistol shrimp (Hess et al, 2013).
Furthermore, Herberholz and Schmitz found a correlation between the pistol shrimp’s claw closure velocity and the velocity of the water jet produced. A claw closure velocity of 5.9 m/s corresponds to a water jet velocity of 6.5 m/s. Moreover, the velocity, distance, and width of the water jet correlates to the pistol shrimp’s claw size. Their overall findings indicate that the larger the claw size, the greater the distance and width of the water jet produced. Continued research by this team also led to the discovery that the cavitation bubbles formed are specifically generated by the tensile force of the water jet. The deafening sound generated by the violent collapse of the cavitation bubbles serves as an indicator of the energy released. The sound — considering sound is a byproduct of energy release (like heat in other cases) — indicates that the cavitation phenomenon can release enough energy to blast, stun, or even kill prey for the pistol shrimp. Or in the case of amphipods, the energy released by the cavitation bubbles collapsing would be strong enough to deter predators (Hess et al, 2013).
If we analyze the mechanism of the dactyl closing into the propodus in more depth, we can model the snapping motion as a spring-loaded lever, as shown in Figure 21.

Fig. 21. Mechanism of the pistol shrimp’s claw snapping [Au, W., & Banks, K., 1998].
In preparing for its claw’s powerful snap, the pistol shrimp will cock the dactylus, as if against a spring, locking it in place. To execute its snap, the pistol shrimp will unlatch the “spring-loaded” dactylus to drive it against the adjacent stationary propodus. In scrutinizing this motion, one observes a time lapse t of approximately 290-300 µs occurring between the moment when the dactylus initially unlatches and when it connects with the propodus to conclude the snap. This time lapse also describes the time it takes for the plunger to fix into the socket. Knowing this, we can estimate the acceleration, force, and velocity involved in a snap. From data collected through experimentation, the average pistol shrimp claw length is measured to be roughly 6.7 ± 0.8 mm. The separation distance between the dactylus and propodus when the former is cocked is measured to be about 2 mm. Therefore, the angle of separation o is measured to be approximately 0.303 rad and the arc length of separation between the tips of the dactylus and propodus s is measured to be approximately 2.03 mm. For the tip of the snapping dactylus, the angular velocity can be expressed by the equation

Eq. 3 Equation for angular velocity.
and the angular acceleration can be expressed by the equation

Eq. 4 Equation for angular acceleration.
Assuming constant acceleration of the tip of the dactylus, we can use Equation 3 and Equation 4 to derive that the angular displacement can be expressed by the equation

Eq. 5 Equation for angular position.
in which C is the constant of integration. At t=0 µs, θ=o rad, so C=o rad. At time t=290 µs, θ=0 rad, so using a rearranged version of Equation 5 we find that α=-7.2×106 rad/s2. The linear acceleration a can be expressed by the equation

Eq. 6 Equation for linear acceleration.
Since r=so=6.7 mm/rad, using Equation 6 we find that a=-48×106 mm/s2 =-48×103 m/s2. The negativity of the linear acceleration of the tip of the dactylus indicates that it occurs in a downward direction. Knowing acceleration due to gravity is –9.81 m/s2, we find that the acceleration of the dactylus snapping against the propodus is approximately 4800 times that amount.
From data collected through experimentation, the average pistol shrimp claw mass m is measured to be roughly 1.6 × 10-6 kg. The force of the dactylus against the propodus F can be expressed by the equation

Eq. 7 Equation for force.
Therefore, using Equation 7, F=0.08 N.
The average linear velocity v can be expressed by the equation

Eq. 8 Equation for average linear velocity.
Therefore, using Equation 8, v=7 m/s (Au, W., & Banks, K., 1998).
As aforementioned, amphipods possess the same claw characteristics as pistol shrimp, shown in Figure 22, and execute the same mechanism to create cavitation bubbles and their resulting shock waves for defense. What distinguishes the amphipod use of these phenomena is their ability to repeatedly snap their claws. The sheer dexterity that the act of repeated ultra-fast snapping requires elevates amphipods to a league of their own. Although much quicker movements made by other marine animals have been discovered, none could be so rapidly repeated as the snaps of the amphipod. The unique repeatability of their quick claw closures makes amphipods remarkable.

Fig. 22. Diagram of the large, dominant claw of amphipods [Longo et al, 2021].
In the in-depth analysis of the claw snapping mechanism, this paper specifically examined the mechanics in their application to pistol shrimp, but the same overall mechanism applies to amphipods. In preparation for snapping its powerful claw, the amphipod will cock the dactylus with an angle of separation measured to be approximately 1.22 rad (as compared to the 0.303 rad angle of separation held by pistol shrimp). Shown in Figure 23, when the amphipod drives the dactylus shut against the adjacent stationary propodus, the time elapsed for this motion is measured to be about 80 µs (as compared to the 290-300 µs snap time of pistol shrimp). Some of the quickest snaps even occurred in less than 50 µs, which is 104 times faster than the average blink of an eye (Longo et al, 2021).

Fig. 23. Speed of amphipod claw closure [Longo et al, 2021].
The dactyl snaps of amphipods, with a speed of approximately 30 m/s (Willis, K., n.d.) and an average linear acceleration of approximately 2.4 × 105 m/s2, rival the maximum linear acceleration of the mandible strikes of mantis shrimp, shown in Figure 24. The significance of comparing amphipod snaps to mantis shrimp strikes lies in the fact that the latter are widely known and have been repeatedly proven to constitute some of the fastest animal movements in nature. Although some terrestrial insects execute faster and even repeatable mandible strikes, this rapid acceleration occurs through air rather than water. Since air is less dense than water, there is less drag and therefore less force required to produce the same value of acceleration as would be produced by organisms such as amphipods and mantis shrimp that experience hydrodynamic drag. This again exhibits just how incredibly fast and forceful amphipod snaps are (Longo et al, 2021).

Fig. 24. Graph of the acceleration of amphipod claw snaps compared to the fastest movements conducted by other animals [Longo et al, 2021].
The researchers of the Patek Laboratory summarize it best: “the fastest motions […] occur in small, obscure creatures that have harnessed three challenges in physics and engineering: (i) extreme acceleration (ii) at small length and mass scales, and with (iii) repeatable and efficient use throughout the life of the organism” (Patek Lab, n.d.). Revisiting one of these creatures, one can consider how instrumental the understanding of the exact mechanisms that make such fast movements possible would be for potentially engineering instruments that could replicate this technique. As such, researchers are analyzing the dynamics that allow mantis shrimp mandible strikes to accelerate as quickly as they do. They have deduced that the ultrafast movements directly correlate to the latching mechanism of the appendages involved in the mandible strikes. The details of this latching system are not yet fully understood, although we can assume a similarity to the amphipod as well as the pistol shrimp snapping mechanisms, as previously described in depth. What researchers do know is that the latching mechanism involves a rapid release of stored elastic energy. Through each distinct phase of this latching mechanism, the mantis shrimp exerts control over the release of energy (Steinhardt et al, 2021). Therefore, knowing the exact system by which the mantis shrimp’s mandible snaps shut becomes essential to recreating the process in an engineered application.
Based on the current although still incomplete knowledge of the mechanisms of mantis shrimp mandible strikes, scientists have engineered models replicating these animals’ movements, even achieving acceleration capabilities of 3.2 × 104 m/s2 to reach velocities of about 25 m/s. Despite their efforts, the capabilities of real mantis shrimp surpass those of commendable engineering, averaging quicker accelerations of 1.5 × 105 m/s2 to reach higher velocities of approximately 30 m/s (Cox et al, 2014). Here one can marvel at how nature continuously transcends feats of engineering; nevertheless, nature serves as an impeccable model for what to strive for.
Conclusion
The sheer versatility and functionality of claws is evident in the varied ways with which animals take advantage of them. It is no wonder that so many varied orders and families of animals have all evolved this powerful tool. From arboreal animals which take advantage of kinetic force applied through claws and angles of penetration from claws used for climbing, to reptiles which use them to support adhesive pads or individually enable locomotion and penetrate prey, to birds who use claws to enable them to perch while also targeting specific prey, to seals which use them for food processing, to pistol shrimps and amphipods which create weaponized water jets with them; the remarkable diversity only serves to display the incredible utility which claws provide. Here, physics plays a role. Passerines achieve something truly remarkable in their perching: compared to inventions like airplanes, which require a long runway to land, passerines use their claw shape to take advantage of surface asperities, surface deformation, and friction forces to land cleanly and efficiently on all sorts of different perches. Phocines, through their strong grasp, also perform admirable locomotion by taking advantage of the traction of their claws to propel themselves forward even on slippery, low-friction, algae-covered surfaces. The study of the physics of these many claw functions furthers one’s understanding of the many ways nature tackles the issues of motion, hunting, resting, and predator intimidation while abiding by its principles of multifunctionality and adaptation. Such understanding can enable biomimetic technology in a variety of fields, from unmanned aerial vehicle perching to climbing equipment, leading to the development of bird claw-inspired grasping mechanisms within unmanned aerial vehicles (Roderick et al., 2021). The comprehension of these physical mechanisms, refined by evolution over millennia, enables engineers to also utilize the methods and solutions which nature has honed over millennia.
References
Alibardi, L. (2020). Development, structure, and protein composition of reptilian claws and hypotheses of their evolution. Anat Rec, 304, 732 757. https://doi.org/10.1002/ar.24515
Allen, N. (2020, August 17). We asked a marine biologist to explain pistol shrimp powers. Vulture. https://www.vulture.com/2020/08/how-powerful-is-a-pistol-shrimp-a-marine-biologist-explains.html
Ashton, T. J., Michie, I., & Johnston, I. A. (2010). A Novel Tensile Test Method to Assess Texture and Gaping in Salmon Fillets. Journal of Food Science, 75(4), S182-S190. https://doi.org/10.1111/j.1750-3841.2010.01586.x
Au, W., & Banks, K. (1998). The acoustics of the snapping shrimp Synalpheus parneomeris in Kaneohe Bay. The Journal of the Acoustical Society of America, 103, 41-47. https://doi.org/10.1121/1.423234
Block, B. (2022, May 6). Bald eagle. Audubon. Retrieved September 23, 2022, from https://www.audubon.org/field-guide/bird/bald-eagle
Cox, S. M., Schmidt, D., Modarres-Sadeghi, Y., & Patek, S. N. (2014). A physical model of the extreme mantis shrimp strike: Kinematics and cavitation of Ninjabot. Bioinspiration & Biomimetics, 9(1), 016014. https://doi.org/10.1088/1748-3182/9/1/016014
Engineering ToolBox, (2005). Mass Moment of Inertia. [online] Available at: https://www.engineeringtoolbox.com/moment-inertia-torque-d_913.html [Accessed 23 Sep. 2022].
Evans, A. R., Pollock, T. I., Cleuren, S. G., Parker, W. M., Richards, H. L., Garland, K. L., Fitzgerald, E. M., Wilson, T. E., Hocking, D. P., & Adams, J. W. (2021). A universal power law for modelling the growth and form of teeth, claws, horns, thorns, beaks, and shells. BMC Biology, 19(1). https://doi.org/10.1186/s12915-021-00990-w
Feduccia, A. (1993). Evidence from claw geometry indicating arboreal habits of archaeopteryx. Science, 259(5096), 790–793. https://doi.org/10.1126/science.259.5096.790
Fowler, D. W., Freedman, E. A., & Scannella, J. B. (2009). Predatory functional morphology in Raptors: Interdigital variation in talon size is related to prey restraint and immobilisation technique. PLoS ONE, 4(11). https://doi.org/10.1371/journal.pone.0007999
Garrett, J. N., & Fish, F. E. (2015). Kinematics of terrestrial locomotion in harbor seals and gray seals: Importance of spinal flexion by amphibious phocids. Digital Commons @ West Chester University | West Chester University Research. https://digitalcommons.wcupa.edu/cgi/viewcontent.cgi?article=1027&context=bio_facpub&httpsredir=1&referer=
Helmenstine, A. (2022, July 17). Mohs hardness scale. Science Notes and Projects. Retrieved September 23, 2022, from https://sciencenotes.org/mohs-hardness-scale/
Hess, D., Brücker, C., Hegner, F., Balmert, A., & Bleckmann, H. (2013). Vortex formation with a snapping shrimp claw. PLoS ONE, 8(11), e77120. https://doi.org/10.1371/journal.pone.0077120
Hildebrand, M., Bramble, D., Liem, K., & Wake, D. (1985). Functional vertebrate morphology (Reprint 2014, Vol. 1). HARVARD UNIV Press.
Hocking, D. P., Marx, F. G., Sattler, R., Harris, R. N., Pollock, T. I., Sorrell, K. J., Fitzgerald, E. M., McCurry, M. R., & Evans, A. R. (2018, April 18). Clawed forelimbs allow northern seals to eat like their ancient ancestors. Royal Society Open Science. https://royalsocietypublishing.org/doi/10.1098/rsos.172393
Holden, R. (2022, May 6). Snowy owl. Audubon. Retrieved September 23, 2022, from https://www.audubon.org/field-guide/bird/snowy-owl
Hollie, D. (2021, February 22). Painted Bunting – eBird. eBird. Retrieved September 23, 2022, from https://ebird.org/species/paibun
Ijiri, M., Shimonishi, D., Nakagawa, D., & Yoshimura, T. (2018). New water jet cavitation technology to increase number and size of cavitation bubbles and its effect on pure al surface. International Journal of Lightweight Materials and Manufacture, 1(1), 12-20. https://doi.org/10.1016/j.ijlmm.2018.03.003
Jenkins, F. A. (1974). Claw Grip and Support Diameter. In Primate locomotion (pp. 56–58). essay, Academic Press. https://asknature.org/strategy/sharp-claws-increase-vertical-agility/
Komodo dragon. Smithsonian’s National Zoo. (2019, October 3). Retrieved October 19, 2022, from https://nationalzoo.si.edu/animals/komodo-dragon
Koukouvinis, P., Bruecker, C., & Gavaises, M. (2017). Unveiling the physical mechanism behind pistol shrimp cavitation. Scientific Reports, 7(1). https://doi.org/10.1038/s41598-017-14312-0
Labonte, D., & Federle, W. (2015). Scaling and biomechanics of surface attachment in climbing animals. Philosophical Transactions of the Royal Society B: Biological Sciences, 370(1661), 20140027. https://doi.org/10.1098/rstb.2014.0027
Lammers, A. R., & Zurcher, U. (2011). Static Stability. In Stability during arboreal locomotion. essay, INTECH Open Access Publisher.
Longo, S., Ray, W., Farley, G., Harrison, J., Jorge, J., Kaji, T., Palmer, A., & Patek, S. (2021). Snaps of a tiny amphipod push the boundary of ultrafast, repeatable movement. Current Biology, 31(3), R116-R117. https://doi.org/10.1016/j.cub.2020.12.025
MacDonald, A. (2013, December 13). Pedal or push? New Scientist. https://www.newscientist.com/lastword/mg22029482-600-pedal-or-push/
Marx, F. G. (2018, April 17). Sharp claws helped ancient seals conquer the oceans. The Conversation. https://theconversation.com/sharp-claws-helped-ancient-seals-conquer-the-oceans-92828
Pacheco, A. (2022, May 6). Peregrine falcon. Audubon. Retrieved September 23, 2022, from https://www.audubon.org/field-guide/bird/peregrine-falcon
Palci, A., Hutchinson, M. N., Caldwell, M. W., Smith, K. T., & Lee, M. S. Y. (2020). The homologies and evolutionary reduction of the pelvis and hindlimbs in snakes, with the first report of ossified pelvic vestiges in an anomalepidid (Liotyphlops beui). Zoological Journal of the Linnean Society, 188(2), 630–652. https://doi.org/10.1093/zoolinnean/zlz098
Patek Lab. (n.d.). Principles of ultrafast movement. Patek Lab – Evolutionary Biomechanics. https://pateklab.biology.duke.edu/research/mechanics-of-ultrafast-movement/
Pike, A. V., & Maitland, D. P. (2006). Scaling of bird claws. Journal of Zoology, 262(1), 73–81. https://doi.org/10.1017/s0952836903004382
Poma, E. L. (2010). 10 fierce facts about Komodo Dragons. World Wildlife. https://www.worldwildlife.org/blogs/good-nature-travel/posts/ten-fierce-facts-about-komodo-dragons
Rhett, A. (2021). Want to Lie on a Bed of Nails? Physics Has Your Back. Wired. https://www.wired.com/story/want-to-lie-on-a-bed-of-nails-physics-has-your-back/
Roderick, W. R. T., Chin, D. D., Cutkosky, M. R., & Lentink, D. (2019). Birds land reliably on complex surfaces by adapting their foot-surface interactions upon contact. ELife, 8. https://doi.org/10.7554/elife.46415
Roderick, W. R., Cutkosky, M. R., & Lentink, D. (2021). Bird-inspired dynamic grasping and perching in arboreal environments. Science Robotics, 6(61). https://doi.org/10.1126/scirobotics.abj7562
Rothschild M.B., Bryant B., Hubbard C., Tuxhorn K., Kilgore P. G., Martin L., Naples V. (2013). The Power of the Claw. PLOS ONE. 10(1371), 0073811. https://doi.org/10.1371/journal.pone.0073811
Russell, A. P., & Bauer, A. M. (1988). Ungual asymmetry in the context of pedal symmetry in Ailuronyx (Reptilia: Gekkonidae): modification for an opposable grip. 218, 1-9
Spinner, M., Westhoff, G. & Gorb, S. (2014). Subdigital setae of chameleon feet: Friction-enhancing microstructures for a wide range of substrate roughness. Sci Rep 4, 5481. https://doi-org.proxy3.library.mcgill.ca/10.1038/srep05481
Stefan, C., Martin S. F., & John, A. N. (2014). Ichnology of an Extant Belly-Dragging Lizard—Analogies to Early Reptile Locomotion? Ichnos, 21:1, 32-43, https://doi-org.proxy3.library.mcgill.ca/10.1080/10420940.2013.877006
Steinhardt, E., Hyun, N. P., Koh, J., Freeburn, G., Rosen, M. H., Temel, F. Z., Patek, S. N., & Wood, R. J. (2021). A physical model of mantis shrimp for exploring the dynamics of ultrafast systems. Proceedings of the National Academy of Sciences, 118(33).
Willis, K. (n.d.). Tiny crustaceans’ claws capable of fastest repeatable movements ever seen in marine animals. University of Alberta. https://www.ualberta.ca/folio/2021/02/tiny-crustaceans-claws-capable-of-fastest-repeatable-movements-ever-seen-in-marine-animals.html
Zug, G. R., & Dowling, H. G. (2022). Reptile. Encyclopedia Britannica. https://www.britannica.com/animal/reptile/Size-range