Abstract

This essay examines the drag-reductive properties of sharks’ scales and analyzes the macroscale and microscale structures and mechanisms that diminished turbulence for the fish in motion over the course of evolution. Fluid dynamics theory and Reynold’s number are used to explain the high reduction of turbulence and friction drag in water by shark scales. It is discussed that the drag reduction effect created by the specific structure of placoid scales and their distribution on the shark’s body benefits the anti-drag effect of shark skin, reducing it by up to 10 %. The antifouling effect reducing drag on shark skin due to scale structure and mucus is also discussed. Finally, the erection of riblets (bristling) is a factor theorized to reduce drag by actively and passively redirecting the flow of water and reducing the turbulence of water to benefit the shark’s movement speed. These mechanisms used to achieve drag reduction are very advantageous and have been studied and replicated for various innovations in biomimicry.

Introduction

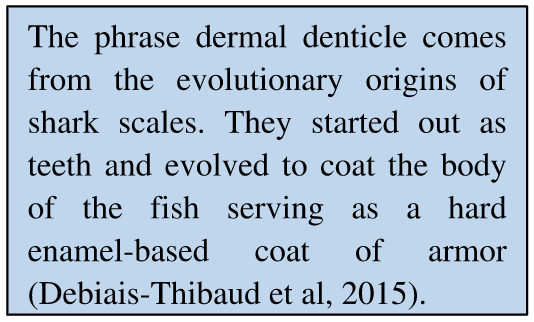
Sharks have existed in a similar form for over 350 million years. Throughout this long history, sharks have preyed on a huge variety of aquatic animals. The exact niches sharks have occupied have shifted along with changes in the earth’s oceans and sharks’ diversification as an order. They have been under constant evolutionary pressure to successfully capture their prey. Sharks have developed numerous remarkable characteristics for survival from the enormous size of the whale shark to the unique head shape of the hammerhead. As a result, their unique scale morphology is sometimes overlooked despite their interesting and valuable effects on the hydrodynamics of shark movement. These scales or dermal denticles contain ridges that reduce turbulence created by the swimming of the shark making their movement quieter and more efficient. This unique scale structure also facilitates movement by displaying antifouling properties that prevent barnacles or other opportunists that could slow the shark’s movement from properly attaching. These scales can also respond to the environment around them by bristling them to reduce drag while swimming. These properties have also been used to inspire biomimetic improvements to many engineering problems.

A general view on drag reduction by shark scales

Before explaining the shark scale’s role in reducing drag, we need to understand some basics of hydrodynamics. Objects moving underwater will mainly face two types of drag, which are pressure drag and friction drag (Dean & Bhushan, 2010). Pressure drag, or form drag, is caused when the liquid flowing against the object requires energy to pass along the object (Dean & Bhushan, 2010). As the fluid partially absorbs the kinetic energy of the object, the object’s velocity decreases, equivalent to the object experiencing a drag bringing an acceleration in the direction opposite to the object’s motion. However, the streamlined shape of the shark body highly reduces the pressure drag, making this drag not a major factor affecting the shark’s motion (Dean & Bhushan, 2010). Hence, friction drag is the external force that the shark mainly faces. The formation of viscous drag or friction drag is due to the attractive interaction among atoms between the fluid and the surface parallel to the flow, as well as among liquid molecules (Dean & Bhushan, 2010). Equivalently, the higher the liquid viscosity (stronger interactions among liquid molecules), the stronger the drag (Dean & Bhushan, 2010). It can be imagined as multiple stacked postal stamps sliding on a block of partially flat ice. The liquid interaction is like the friction between stamps, while the interaction between liquid and surface is like the friction between stamps and ice. Due to the drag, if the flow is divided into layers away from the surface, the flow velocity gradually increases from the surface to the more exterior layers until the mean velocity of the fluid is reached (Dean & Bhushan, 2010). In other words, the friction drag can be explained as a result of momentum transfer between undisturbed fluid and the shark’s surface parallel to the flow, causing the velocity difference amongst the different layers (Dean & Bhushan, 2010).

The friction drag can be affected by the complex patterns of flows. The ratio of inertial force (created by the fluid’s momentum) to the frictional force of the fluids can be defined as the Reynolds number (Re) (Dean & Bhushan, 2010). The equation for the Reynold number can be written as

\begin{equation}
Re=\frac{\rho VL}{\mu}
\end{equation}

(Dean & Bhushan, 2010). In this equation, ρ stands for the liquid density, V is the mean velocity of the fluid, L is the diameter in pipes and length in flat plates, and μ stands for fluid viscosity (Dean & Bhushan, 2010). When the Reynolds number is low, laminar flows form because the frictional force dominates, while turbulent flows lead to a higher Reynolds number since inertial force dominates (Dean & Bhushan, 2010). Particles in laminar flows move in ordered smooth layers, while those in turbulent flows move in different pressure and velocity vectors, including some cross and unparalleled flows (Batchelor, 2000). Usually, the boundary between laminar and turbulent flows occurs in the Reynold numbers of about 4000 in pipes and approximately 500,000 over flat plates (Dean & Bhushan, 2010). The fast-swimming sharks swim at high speeds, causing the Reynold numbers for fluid near the shark’s skin to be usually high, leading to frequent turbulent flows in the viscous sublayer near shark scales. For an object with a flat surface, the chaotic liquid motion due to turbulent flows in the viscous sublayer near the surface can form a cross-movement of liquid, creating vortices (Dean & Bhushan, 2010). As more vortices appear and interact with each other, an outward bursting will occur, causing more chaotic motions in the viscous sublayer (Dean & Bhushan, 2010). It can even create an increasing number of vortices and bursting events. Collisions between vortices and between vortices and the object’s surface will result in momentum transfer from the object, thus causing a drag on it (Dean & Bhushan, 2010).

The role of shark scales is mainly to reduce the effect of chaotic motion near the scale’s surface. The tiny riblets (see Figure 1) on the shark’s scale can stop the cross-stream fluid translation of vortices, effectively eliminating vortices’ occurrences and reducing the shark’s momentum loss (Dean & Bhushan, 2010). However, the exact mechanisms behind it are still a vacant space for research nowadays (Dean & Bhushan, 2010). Some research shows that the drag reduction only exists in the turbulent flow region instead of the laminar one, and it is shown in Figure 2 (Fu et al., 2017). Around this finding, some rough explanations for drag reduction by the shark scales are established. Based on Figure 3, it is observed that when vortices appear over the scale surface, they will only stay over the riblets’ surface, meaning the chaotic motions of fluid only interact with the tips (Dean & Bhushan, 2010). It seldom causes the high-speed flow in the valley between two shark riblets (Dean & Bhushan, 2010). According to the formation of drag mentioned above, the faster the liquid molecule, the more collisions happen among molecules, transferring more momentum from the object and forming more drag on it. Thus, only shark scales’ tips experience much stronger shear stresses or friction drag than other scale regions only facing low-speed flow (Fu et al., 2017). This can also be reflected in Figure 2. In the case of shark scales, strong friction forces due to turbulent flow are only on riblets’ tips. Most of the scale body gets a comparatively low drag, therefore highly reducing the drag all over the shark body (Dean & Bhushan, 2010). Some specific characteristics, such as scale geometry, distribution, antifouling effect, and bristling of shark scales, play roles in reducing the overall drag, which will be discussed later.

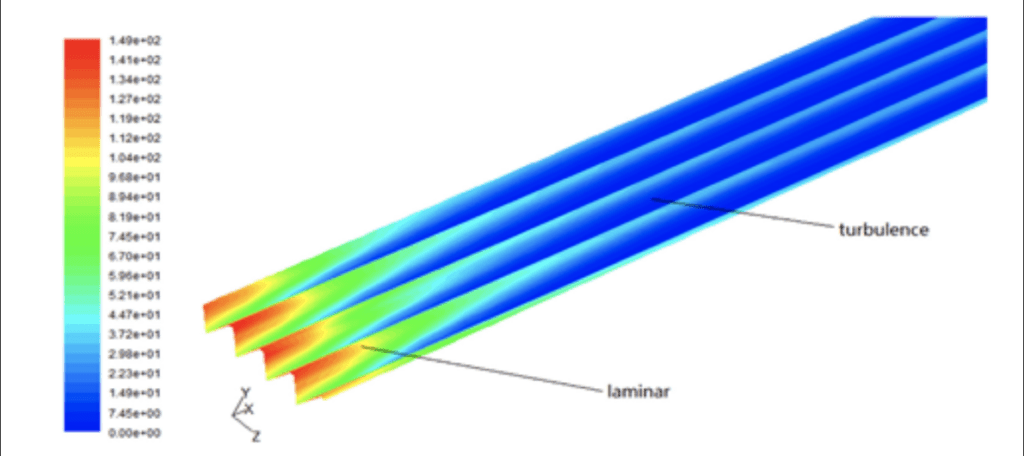
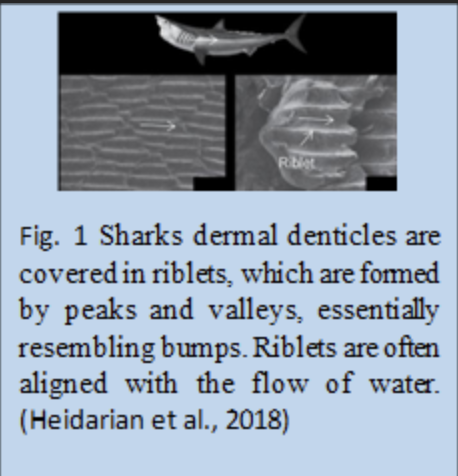
Fig. 2. Visualization of shear stress on the riblet regions and smooth surfaces in various areas of flows. The drag reduction mainly occurs in the turbulence area instead of the laminar one (Fu et al., 2017). 

Fig. 3. Visualization of vortices caused by turbulent flows in the vertical cross-section over a flat plate and riblets (Dean & Bhushan, 2010). The “s” stands for riblet spacing, while the “h” means the riblets’ height (Dean & Bhushan, 2010). “+” is a dimensionless wall unit (Dean & Bhushan, 2010). Pictures (a) and (c) show the case when the flow speed is 3m/s (comparatively low drag), while the pictures (b) and (d) present the case of flow speed of 5 m/s (comparatively high drag) (Dean & Bhushan, 2010). It is shown that the vortices by the turbulent flows stay mainly over the riblets’ tips. 

Characteristics of Riblets and Their Role in Drag Reduction 

Although it may be apparent that there is a variation in scales between different shark species, scales also vary drastically within the same specimen. A scale can be characterized by its crown length and width, and riblet spacing and depth, as shown in Figure 4.

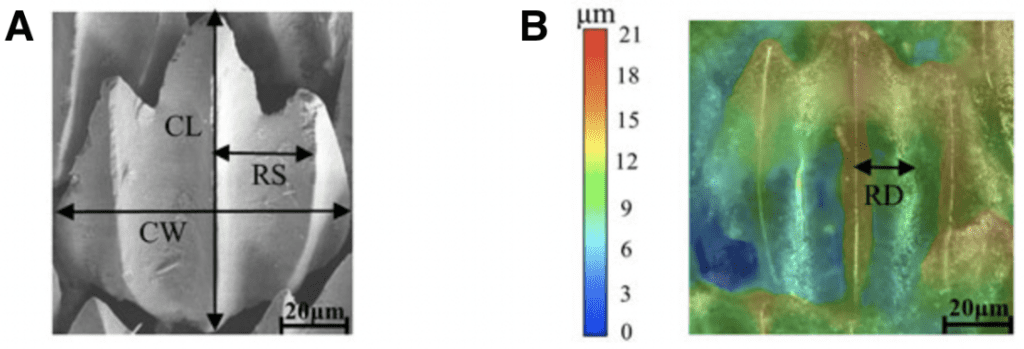
Fig. 4 Riblet structure and shape. (A) shows where the crown length (CL), crown width (CW), and riblet spacing (RS) are measured. (B) shows how the riblet depth (RD) is measured using a color gradient (modified from Chengchun et al., 2022).

The crown length on riblets of fast-swimming sharks normally measures between 180 and 550 µm (Lang et al., 2012). On the fast-swimming mako shark, the larger scales, thereby the ones with the larger crown length and width, are found on the leading edge of the pectoral fin (Chengchun et al., 2022). The crown length of these fins measures about 286 µm, and the width about 255 µm (Chengchun et al., 2022). The smallest crown length and width found on the mako shark measure around 105 and 87 µm, respectively, and are found on the trailing edge of the shark’s tail (Chengchun et al., 2022). On pectoral, dorsal, and caudal fins, it can be observed by looking at measurements of the crown length and width in Figure 5 with their corresponding body parts in Figure 6 that there is a tendency for the leading edge of a fin to be composed of larger riblets than the trailing edge of the same fin. The leading edges of these fins are highlighted in yellow, while the trailing edges are in blue. It is unclear what the relationship between the scale’s dimensions and drag reduction is, but the characteristics of the riblets themselves clearly impact drag reduction.

Fig. 5 Measurements of the riblets in various parts of the mako shark’s body. The corresponding body parts for each measurement can be found in Figure 6 (modified from Chengchun et al., 2022).

Fig. 6 Body parts of the mako shark where the measurements were taken for Figure 5 (modified from Chengchun et al., 2022).

Riblets significantly differ within the same shark specimen, as is depicted in Figure 7. Indeed, characteristics such as the height, the spacing, and the cross-sectional structure of riblets differ depending on where they are located on the shark’s body in order to efficiently reduce drag (Chengchun et al., 2022).

Fig. 7 Difference in riblet morphology depending on their location on the mako shark (Isurus oxyrinchus) (Chengchun et al., 2022).

There is a strong correlation between the flow field at a particular location of the shark’s body and the characteristics of the riblets at that same location. For instance, the pressure at a particular location on the shark skin affects the height of the riblets. The difference in height in riblets due to their location can be explained by the fact that riblets majorly reduce drag in turbulent areas and less in laminar areas (Fu et al. 2017). The riblets with the greatest height are located where there is less wall shear stress, which is in a turbulent flow area. Oppositely, the flatter scales are located on leading edges where there are more wall shear stress, in a laminar flow area. For example, scales located on the nose and the leading edges of fins tend to be smoother because they are subject to greater shear wall stress compared to scales located elsewhere, which are composed of well-defined riblets. (Chengchun et al., 2022). The body parts facing water flow and under more pressure can be visualized in Figure 8.

Fig. 8 Representations of the pressure and velocity around the shark’s body (Chengchun et al., 2022).

The density of scales is a factor that varies depending on location and flow field. Compared to other body parts in the mako shark, those which directly face the flow of water, like the nose and leading edge of fins, experience more pressure. The scales in those areas are smoother, less dense, less stiff, and tougher. Oppositely, regions of the mako shark’s body that do not directly face the flow of water, as is the case for the shark’s back, are faced with lower pressure. The scales in that region are denser, stiffer, and less tough (Chengchun et al., 2022; Creager & Porter, 2018). 

Moreover, the spacing between two adjacent riblets is a crucial aspect of reducing drag; riblets must be properly spaced out to reduce drag efficiently. Riblets that are adequately spaced out raise the streamwise vortices created in the turbulent flow, as explained previously, which keeps the vortices on the riblet’s tips and away from the riblet valleys at the surface of the shark. Thus, the tips of the riblets are the parts experiencing the most shear stress and consequently reduce drag in riblet valleys on the surface of the shark’s body. It is important to note that the riblets lift the main vortices away from the riblet valleys, but there are still some smaller vortices that can form in the valleys. However, the shear wall stress caused by these smaller vortices is relatively small (Martin & Bhushan, 2014). There are two other scenarios in which the riblets are not properly spaced out and do not successfully reduce drag. The first case is the one where the riblets are too close to each other. Under this circumstance, there would be more riblets in a given area, increasing the surface area of the shark in contact with vortices in the turbulent flow (Martin & Bhushan, 2014). This increase in the area in contact with vortices results in an increase in drag (Bixler & Bhushan, 2013). This would defeat the riblets’ role, which is to raise the vortices to make the shark’s skin be as little as possible exposed to these vortices, as the vortices would encounter more riblet tips than necessary. The second case is if the riblets are too spread out from one another. In this case, the riblet peaks cannot effectively reduce drag by raising the vortices away from the riblet valleys. The vortices would be in contact with the valleys, as the distance from the peaks is too large, which increases drag (Martin & Bhushan, 2014; Bixler & Bhushan, 2013).

The number of riblets on a given scale also depends on its location across the shark’s body, and the number of riblets per scale varies between species. For instance, most scales on the mako shark possess three riblets, but the slow-swimming blacktip shark has five riblets per scale (Chengchun et al., 2022; Lang et al., 2012). 

Also, the geometry of the riblet affects its ability to reduce drag. In fact, the shape of riblets varies between species and throughout the same shark specimen, depending on their location. The cross-sectional shape of a riblet falls into one of the following categories: blade, scalloped, sawtooth, or trapezoidal. These various shapes can be seen in Figure 9. While many other shapes could describe a riblet’s geometry, such as riblets with peaks that are rounder, only the categories mentioned offer a significant advantage in reducing drag (Dean, 2010).

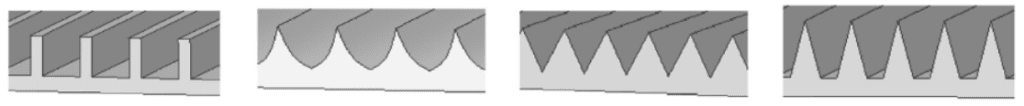
Fig. 9 Different cross-section geometry of riblets. From left to right: blade, scalloped, sawtooth, trapezoidal (Soleimani & Eckles, 2021).

The ratio between the height of a riblet and the spacing between two adjacent riblets, which is referred to as the h/s ratio, varies depending on the riblet’s cross-sectional shape. Some cross-sectional shapes are better at reducing drag because they have an h/s ratio that is more favorable to maintain vortices away from the shark’s skin, which in turn reduces drag, as explained previously. When different riblet shapes were modeled and analyzed, the ideal h/s ratio found for the blade shape was equal to 0.5. This shape can reduce drag by up to 10%. Moreover, thinner riblets of blade geometry further reduce drag. The scalloped shape comes second, as it reduces drag by 6.5% and has an h/s ratio of 0.7. The sawtooth is, therefore, one of the less ideal shapes, with an h/s ratio of 1 and a drag-reducing effect of 5% (Dean, 2010). The trapezoidal shape has similar drag-reducing effects to the sawtooth shape (Soleimani & Eckles, 2021). Even though the blade geometry offers the best drag-reducing results, it is less durable and the hardest to recreate (Fu et al., 2017). The trapezoidal shape is the most durable, followed by the sawtooth, scalloped, and blade shapes, respectively (Lang et al., 2012). 

There is also a difference in the configurations of the riblets, as shown in Figure 10. The continuous configuration is the least efficient in reducing drag, as there is more surface area that is in contact with the high-speed flow of water, known as the wetted area. The two segmented configurations are, therefore, the best at reducing drag (Fu et al., 2017).

Fig. 10 The three configurations of riblets: continuous, aligned segmented, and staggered segmented (Fu et al., 2017).

The cross-section geometry of riblets varies a lot on the same shark specimen. However, on most sharks, the riblets are similar to the scalloped shape that is staggered segmented. Also, the ratio h/s on sharks is often seen to be 0.5, which was considered the ideal ratio when riblets were modeled (Bixler & Bhushan, 2013; Lang et al., 2012). This all demonstrates that riblet structure is not arbitrary but rather has a significant impact on sharks’ ability to reduce drag.

Furthermore, there is a broad range of applications for the shark scales’ mechanism. During the 1980s, vinyl-film riblets on the boat hull are designed for racing competitions, and this technology is utilized in sailing yachts in America’s Cup and even rowing boats in the Olympics (Dean & Bhushan, 2010). As about 48 percent of the total drags on an airplane are skin friction, many researchers seek a biomimetic application of shark riblets for reducing the drag, and the vinyl-film riblets are applied to test airplanes in the Boeing and the Airbus (Dean & Bhushan, 2010). Although the riblets’ biomimetic technology has not yet been applied to commercialized airplanes, it has great potential in the future of aeronautical engineering since the riblet’s drag reduction can help air companies save costs, such as fuel costs (Dean & Bhushan, 2010). There is research showing that if the riblets cover 70 percent of the airplane body, 3 percent of drag reduction can be achieved, saving approximately 3 percent of fuel costs (Dean & Bhushan, 2010). The riblets even appear in commercialized pipes. In experiments, the designs of riblet-shape roughness can reduce fluid drag by 10 percent with extra cleaning effects (Dean & Bhushan, 2010). It has been proven by commercial operations on a 16 km gas-pipeline section with this design (Dean & Bhushan, 2010).

Furthermore, the most commercialized application on the riblets is biomimetic swimwear (Dean & Bhushan, 2010). The most well-known one is the Fastskin swimsuit designed by Speedo in 2004 (Dean & Bhushan, 2010). According to Figure 11, the surface of that swimwear is woven with some tiny grooves on the top and shapes like blade riblets, resembling the riblets on sharks (Dean & Bhushan, 2010). However, there are still some difficulties in manufacturing this type of swimwear by machines, as current standard machine weaving does not have enough flexibility to produce these intricate riblets (Dean & Bhushan, 2010). To solve that problem, weaving technologies, such as assembling separately fabricated blade parts and rolling methods by using a grinding wheel to press the objects into riblet designs, are studied (Dean & Bhushan, 2010).

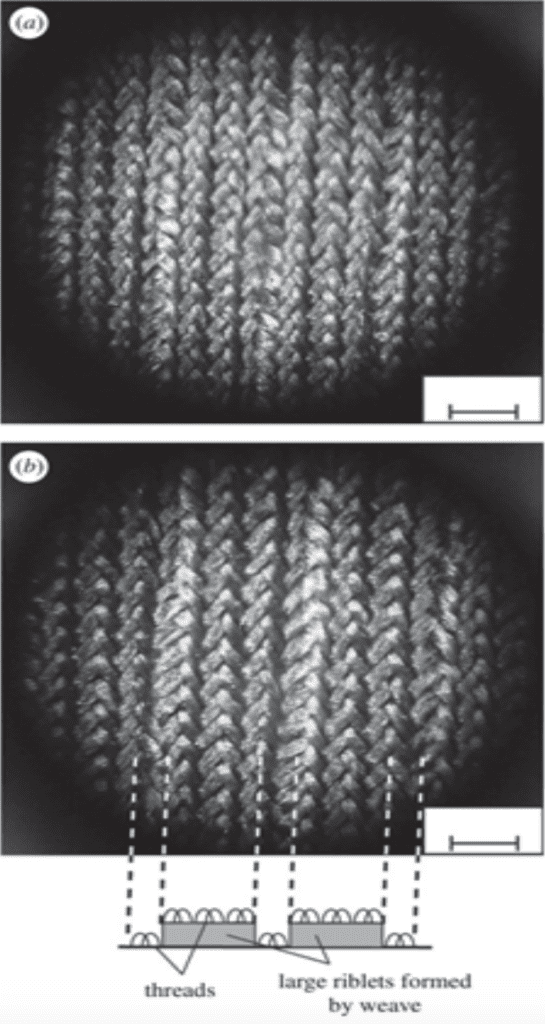
Fig. 11 Images of riblets designs on the biomimetic swimwear (a) a surface photo of the swimsuit; (b) a brief description of the swimwear’s riblet-shape surface (Dean & Bhushan, 2010).

Antifouling

As the characteristic geometry of scales affects the hydrodynamics of shark movement, another contributing factor is the antifouling effect of shark scales. 

Biological fouling is the attachment and accumulation of living organisms on a host surface, such as another living organism or an object. Biofouling can occur on the scale of microorganisms, which accumulate to form biofilms on the surface, or on a macroscale (Bixler & Bhushan, 2012). During this process, the bio-adhesion of microalgae and bacterial biofilms can occur on the surface and can be followed by the accumulation of larvae, macroalgae, mussels, and barnacles on the host (Mo et al., 2021). Such an accumulation of organisms of varying dimensions on the surface of a shark’s body would augment the drag force exhibited on the shark. Thus, the antifouling mechanisms of shark scales are essential to maintain minimum drag for this apex predator (Chien et al., 2020). 

One of these mechanisms is put in place by the structure of shark scales. In general, surfaces exhibiting smoothness are more likely to accumulate microorganisms which can form biofilms on the surface (Schumacher et al., 2007). Shark denticles have a topological nanostructure that is incompatible with the settlement of biofilms, which have a defined structure composed of microbial cells and an extracellular polymeric substance matrix (Chien et al., 2020; Donlan, 2002). An example of a biofilm structure can be visualized in Figure 12. The small ridges of shark denticles counteract the attachment of biofilms because they reduce the surface available for microorganisms and biofilm structures to settle onto and often have micro-ridges that are smaller than the fouling microorganisms themselves (Chien et al., 2020).

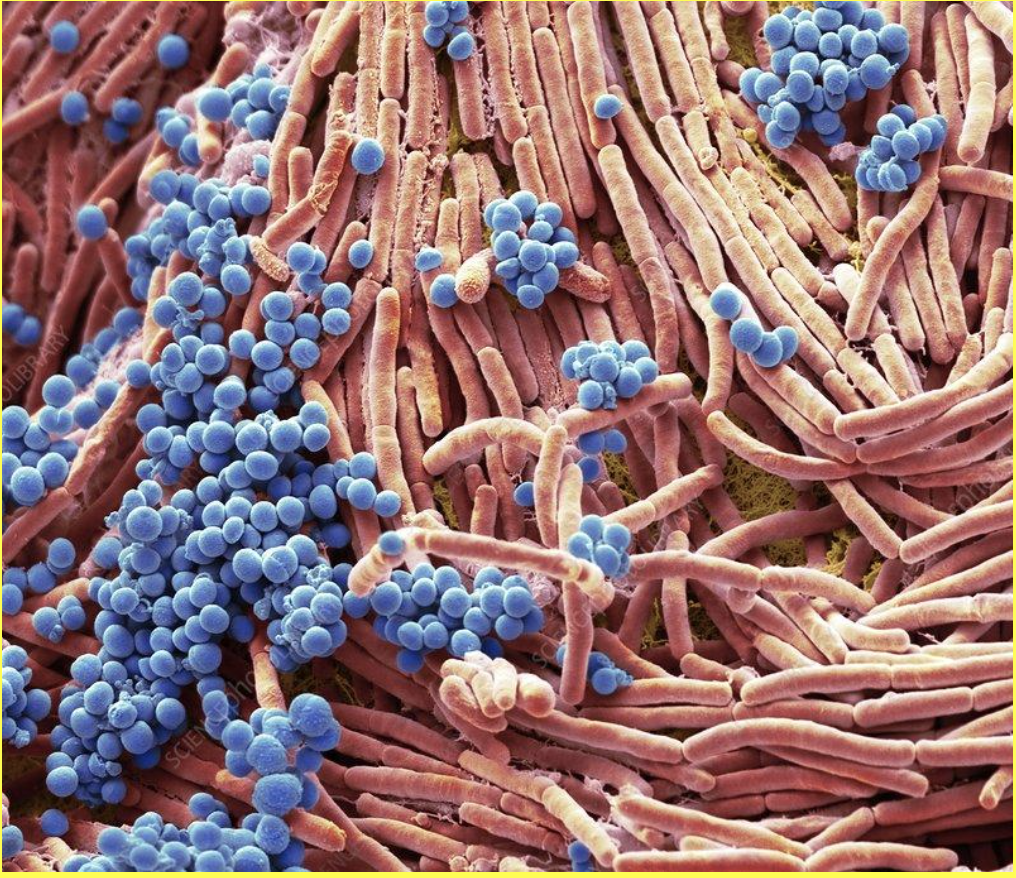
Fig. 12 Colored scanning electron micrograph (SEM) of bacteria cultured on a mobile phone. Magnification: x4000 when printed at 10 cm wide (Gschmeissner).

The second mechanism for antifouling originates from the mucus barrier on shark skin. Fish produce mucus to protect them from external pathogens such as parasites, viruses, algae, bacteria, and fungi with which they come into contact in marine environments (Rakers et al., 2013). This mucus secreted by mucus goblet cells on the skin has antibacterial properties, which prevent the accumulation of bacteria on the surface of the scales, as seen in the schematic image in Figure 13 (Rakers et al., 2013). A protection field is created by the piscine AMPs (antimicrobial peptides) and other antibacterial substances such as alkaline phosphatase, cathepsin B, complement, transferrin, and lysozyme (Rakers et al., 2013). AMPs are a type of peptide that can utilize various mechanisms to destroy microbes. Hepcidin, defensin-like peptides, cathelicidins, apolipoproteins, and pleurocidin are common piscine AMPs found on fish skin (Rakers et al., 2013). These antibacterial, antifungal, and antiviral AMPs use varying mechanisms, such as inhibition of DNA, RNA or protein synthesis and membrane disruption, that attack cell membranes to disarm pathogens on the scales and immunize the animal (Guilhelmelli et al., 2013; Rakers et al., 2013).

Fig. 13 Schematic representation of the epithelial immune defense mechanisms of fish (Rakers et al., 2013). Mucus goblet cells secrete mucus containing AMPs like hepcidin, defensin-like peptides, peuricidin, and pleurocidin. These peptides primarily use inhibition of DNA, RNA or protein synthesis or membrane disruption on pathogens for defense (Rakers et al., 2013).

Moreover, the fast-flowing water near the surface of the shark’s skin dislodges bacteria during the animal’s high-velocity movement at a faster rate than the time required for them to adhere to the surface, thus helping reduce biofouling on the skin surface (Chien et al., 2020). The movement of the scales due to the bristling effect also helps to dislodge organisms which could potentially form biofilms on the skin (Bixler & Bhushan., 2012).

Bristling

The previously described hydrodynamic advantages presented in shark scales focus on the benefits provided by their unique shape and composition; however, novel research has come out examining the active drag reduction capabilities of scales in certain fast-swimming shark species. It is theorized that scales can “bristle” or be erected, allowing them to function as a boundary control mechanism by changing their attack angle (Lang, 2008). Different levels of extension are shown in Figure 15. If all scales were held in this extended position perpetually, they would slow the shark down by increasing the shark’s surface area in water. This would lead to greater overall drag and more force moving in the opposite direction of the sharks’ movement. However, by reactively extending with the side-to-side undulation of the sharks’ swimming, scales on certain areas of the shark would extend and limit turbulence formation, reducing drag and increasing the efficiency and speed of a shark (Lang, 2008). There are still questions surrounding the exact hydrodynamics benefits of bristling due to the extremely high complexity of accurately modeling the flow of water around a living shark. Additionally, the exact mechanism of the scale erection is unclear; however, both passive and active methods seem viable.

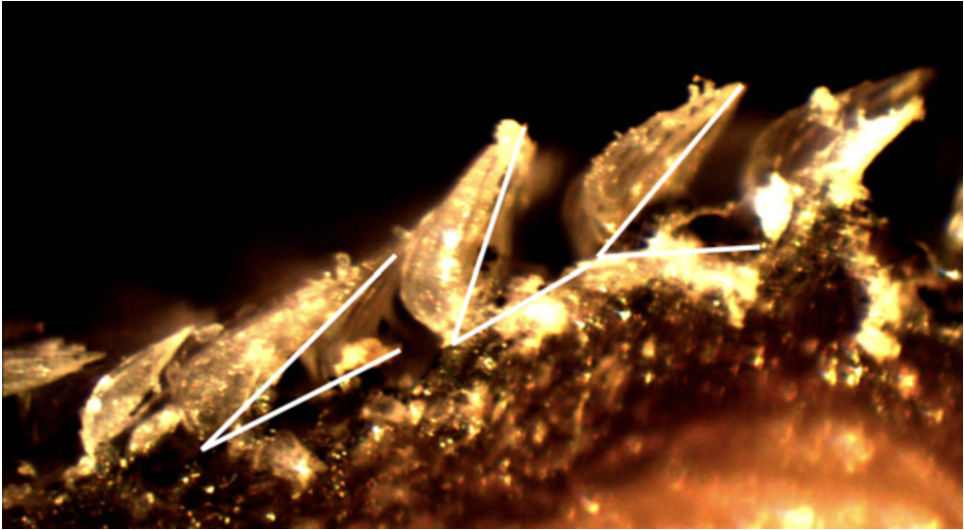
Fig. 15 Images of shark scales at different levels of bristling captured with a dissection microscope at 40x magnification (Lang, 2008).

With higher attack angles, the direction of the vortices created by the scales can be changed. This reduces the drag produced by pushing away certain turbulent bursts away from the body of the shark and preventing them from colliding with the shark’s body and sapping momentum. The vortices created by the scales are illustrated in Figure 16 (Lang, 2008). As shown, each channel in the scale produces a small secondary vortex when in an upright position. When the body of the shark swings back and forth during swimming, water flows collide with the bent portion of the tail. At certain angles, the tail could serve as a sail for these flows, catching them and allowing them to slow the shark down further. By actively redirecting the flow with the vortices created by riblets, this drag can be greatly reduced (Lang, 2008). Quantification of this drag reduction is difficult because, at different angles and speeds, the scales display different hydrodynamic effects, and it is difficult to collect data from swimming sharks to determine which angles are most common. However, with the use of shark models outfitted with movable scales, bristling is shown to have an overall positive effect on swim speed (Du Clos, 2018). Establishing the advantage of this development brings up the question of the exact mechanism that would allow the scales to bristle.

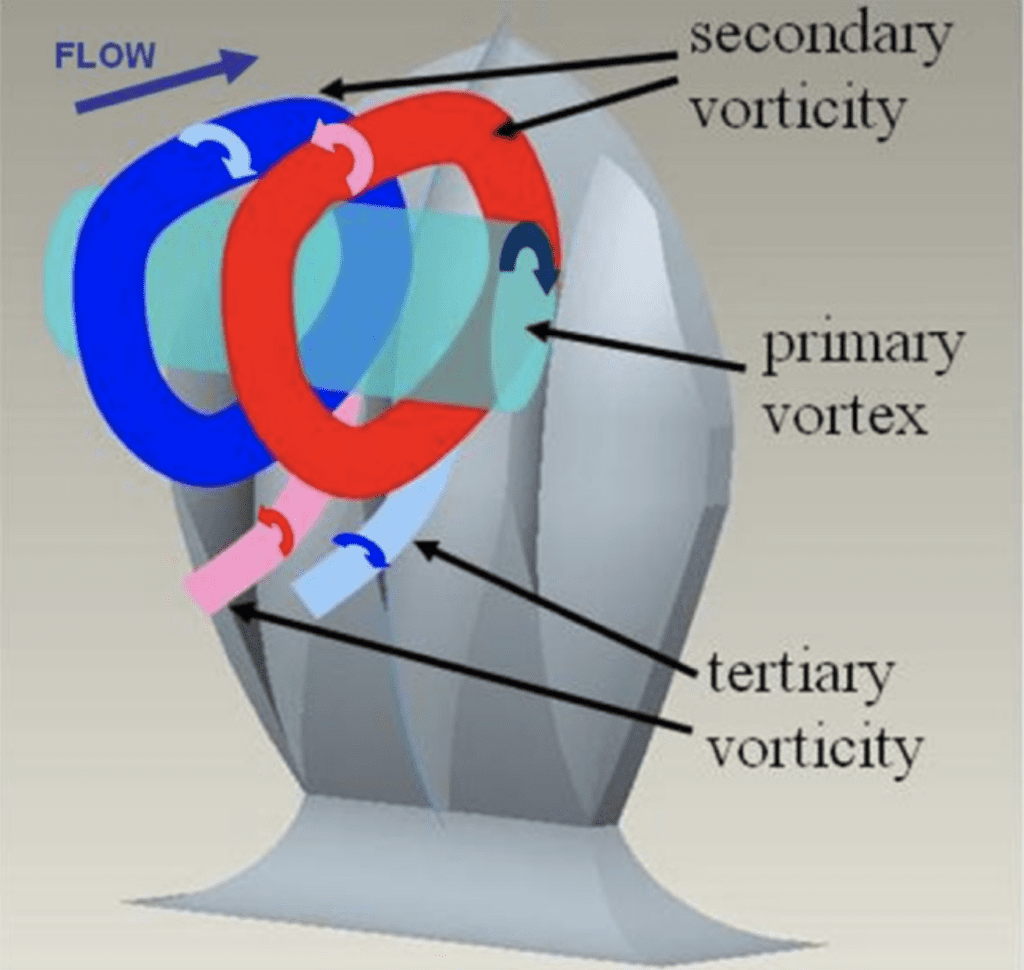
Fig. 16 Diagram of vortices created by modeled shark scales (Lang, 2008).

The scales could be erected to induce this bristling effect either passively or actively. Passive bristling would rely on changes in water pressure and the vortices created by the ridges on the scales to lift the scales (Du Clos, 2018). Active bristling would use the skin of sharks to which the dermal denticles are attached to raise and lower the scales (Lang, 2008). It is important to note that neither form of erection control would be directly connected to the shark’s nervous system. Both theorized methods would change the scale attack angle because of activities the shark would be performing even if the bristling behavior was not present. The simplicity of this system speaks to the long time scales over which sharks have been evolving. There is no wasted motion, energy, or thought on the shark’s part showing the incredible optimization capabilities of evolution.

The dermal denticles of sharks are embedded into deeper layers of skin with high collagen content known as the stratum compactum (Lang, 2008). The stratum compactum consists of interwoven collagen fibers that form a mesh. These fibers are kept pre-stressed to maintain tightness in the skin (Lang, 2008). This additionally allows the layer to store elastic energy while swimming, tightening the individual collagen fibers. The base of the scales is attached to the collagen fibers in this layer (Lang, 2008). As the shark swims, the subcutaneous pressure of the stratum compactum can increase dramatically. This change in pressure leads to a conformational change around the fibers attached to the base of the scale. By altering the base attachment, the crown of the scale that interacts with the water could experience a change in angle, resulting in the scale erection. This was verified by taking recently deceased shark specimens and applying pressure to their skin; bristling behavior was seen in multiple species of sharks with this method (Lang, 2008). 

Alternatively, the scales could be erected by the water flow around the body of the shark. Different pressure gradients and vortices would allow the scales to be elevated. The backflows created by the riblets can push scales up from below. Additionally, the secondary vortex created by the scales also creates a negative pressure region at the tip of the crown that could pull the scale upwards without the need for backflow. Models of different flow patterns of the scale are shown in Figure 17.

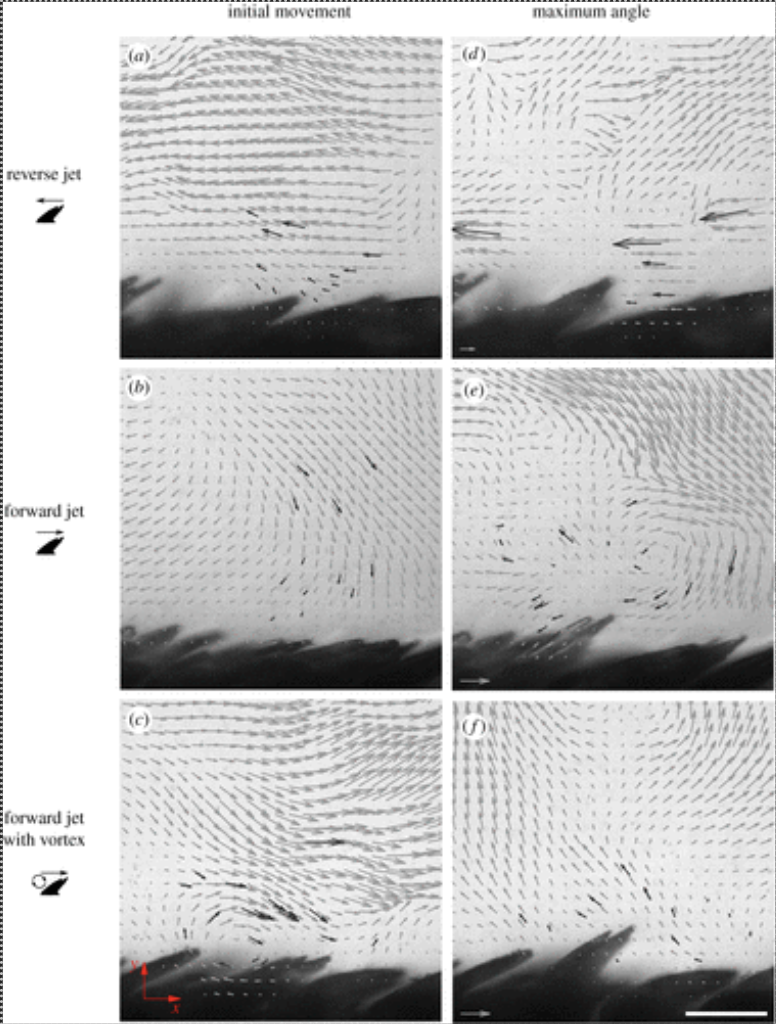
Fig. 17 Effect of different flow patterns on flank scales from a shortfin mako (Du Clos, 2018).

As shown in all cases, the fluid dynamic effects around the scales are sufficient in erecting the scales to a mean maximum angle of at least 33° (Du Clos, 2018). The largest angle easily obtained with external mechanical force on these scales is 30-40° , depending on the region of the shark’s body the scale is selected from (Lang, 2008). This suggests that the hydrodynamic forces alone are strong enough to erect the scales. 

Both bristling control methods appear to be feasible in sharks, so it is likely a combination of both methods is responsible for regulating the erection of scales. This could allow finer control over the bristling angles as the two regulatory methods cooperate to ensure that the drag reduction is optimized. 

Due to the high complexity and specificity of the sharks’ body shape the bristling behavior of shark scales has not been used to develop any biomimetic systems. More research into the turbulence reduction methods is needed to successfully apply the system.

Conclusion

This essay has analyzed the mechanisms granting shark scales highly effective drag-reductive properties. In fact, it is known that this group of fish has evolved in such a way that their scales’ composition and shape grant them a 10% drag reduction as opposed to the drag they would be subject to without the effect of these riblets (Dean, 2010). It was discussed that the shark is subjected primarily to friction drag on the surface of its skin, which is caused by turbulent flows and vortices near the scales. However, the protrusion of riblets interrupts these vortices, thus reducing the turbulent flows near the scales and lowering friction drag (Dean & Bhushan, 2010). 

The distribution of riblets on the animal’s body and the shape of the dermal denticles was discussed to express the importance of these factors on drag reduction. Differences in height, density, and shape of the riblets on the shark’s body affect the efficacy of the reduction of drag due to the interruption of fluid vortices (Chengchun et al., 2022; Martin & Bhushan, 2014). The ratio between the height of a riblet and the spacing between two adjacent riblets is found to be most beneficial at a value of 0.5 (Dean, 2010). Moreover, shark scales have an antifouling effect caused by their shape as well as by the mucus they produce, which prevents the adhesion of biofilms on the skin. This antifouling property eliminates potential sources of increased drag by keeping the scales obstruction-free in the marine environment (Chien et al., 2020). 

Lastly, it is theorized that scales can ”bristle” to adjust their attack angle as the shark moves, thus reducing turbulence formation in certain areas on the surface of the skin (Lang, 2008). The shark may use a combination of active and passive mechanisms to erect its scales for maximum drag reduction. The active method would rely on the collagen-rich dermal tissue in which the denticles are embedded, which tightens with changing water pressure to erect the scales. The passive method utilizes the vortices and water pressure to raise the denticles as the animal swims (Lang, 2008). 

In sum, the mechanisms to maximize the speed and efficiency of the animals’ movement are complex, and the structures involved are efficiently multifunctional. The various characteristics of shark scales work to reduce drag by limiting biofouling, interrupting and redirecting turbulent flows in precise ways on the shark’s body. 

These various successful mechanisms have inspired many applications in biomimicry, such as antibacterial material for medical devices, biomimetic swimwear, and fluid-drag-reducing pipelines (Dean & Bhushan, 2010). The potential for biomimetics based on shark riblets has yet to be fully realized, and exciting applications such as drag-reducing materials used on commercial airplanes continue to be explored by researchers (Dean & Bhushan, 2010).

References:

Batchelor, G. K. (2000). An introduction to fluid dynamics.

Bixler, G. D., & Bhushan, B. (2012). Biofouling: Lessons from nature. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 370(1967), 2381–2417. https://doi.org/10.1098/rsta.2011.0502

Bixler, G.D., & Bhushan, B. (2013). Fluid Drag Reduction with Shark-Skin Riblet Inspired Microstructured Surfaces. Advanced Functional Materials, 23(36), 4507-4528. https://doi.org/10.1002/adfm.201203683 

Chien, H.-W., Chen, X.-Y., Tsai, W.-P., & Lee, M. (2020). Inhibition of biofilm formation by rough shark skin-patterned surfaces. Colloids and Surfaces B: Biointerfaces, 186. https://doi.org/10.1016/j.colsurfb.2019.110738

Chengchun, Z., Gao, M., Liu, G., Zheng, Y., Xue, C., & Shen, C. (2022). Relationship Between Skin Scales and the Main Flow Field Around the Shortfin Mako Shark Isurus oxyrinchus. Frontiers in Bioengineering and Biotechnology, 10. https://doi.org/10.3389/fbioe.2022.742437 

Creager, S. B., & Porter, M. E. (2018). Stiff and tough: a comparative study on the tensile properties of shark skin. Zoology, 126, 154-163. https://doi.org/10.1016/j.zool.2017.10.002

Dean, B., & Bhushan, B. (2010). Shark-skin surfaces for fluid-drag reduction in turbulent flow: A review. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 368(1929), 4775-4806. https://doi.org/10.1098/rsta.2010.0201

Debiais-Thibaud, M., Chiori, R., Enault, S., Oulion, S., Germon, I., Martinand-Mari, C., & Borday-Birraux, V. (2015). Tooth and scale morphogenesis in shark: an alternative process to the mammalian enamel knot system. BMC Evolutionary Biology, 15(1), 1-17.

Donlan, R. M. (2002). Biofilms: Microbial Life on Surfaces. Emerging Infectious Diseases, 8(9), 881–890. https://doi.org/10.3201/eid0809.020063

Dundar Arisoy, F., Kolewe, K. W., Homyak, B., Kurtz, I. S., Schiffman, J. D., & Watkins, J. J. (2018). Bioinspired Photocatalytic Shark-Skin Surfaces with Antibacterial and Antifouling Activity via Nanoimprint Lithography. ACS Applied Materials & Interfaces, 10(23), 20055–20063. https://doi.org/10.1021/acsami.8b05066

Du Clos, K. T., Lang, A., Devey, S., Motta, P. J., Habegger, M. L., & Gemmell, B. J. (2018). Passive bristling of mako shark scales in reversing flows. Journal of The Royal Society Interface, 15(147).

Fu, Y., Yuan, C., & Bai, X. (2017). Marine drag reduction of shark skin inspired riblet surfaces. Biosurface and Biotribology, 3(1), 11-24. https://doi.org/10.1016/j.bsbt.2017.02.001

Guilhelmelli, F., Vilela, N., Albuquerque, P., Derengowski, L. da S., Silva-Pereira, I., & Kyaw, C. M. (2013). Antibiotic development challenges: The various mechanisms of action of antimicrobial peptides and of bacterial resistance. Frontiers in Microbiology, 4(353). https://doi.org/10.3389/fmicb.2013.00353

Heidarian, A., Ghassemi, H., & Liu, P. (2018). Investigation the Effects of Micro-Riblet Film on a Wing-in-Ground Effect. International Journal of Multidisciplinary Sciences and Engineering, 9(5), 8-14. https://www.researchgate.net/publication/325659412_Investigation_the_Effects_of_Micro-Riblet_Film_on_a_Wing-in_Ground_Effect 

Genzer, J., & Efimenko, K. (2007). Recent developments in superhydrophobic surfaces and their relevance to marine fouling: A review. The Journal of Bioadhesion and Biofilm Research, 22(5), 339-360. https://www.tandfonline.com/doi/full/10.1080/08927010600980223 

Gschmeissner, S. Bacteria found on mobile phone, SEM [Photograph]. Science Photo Library. https://www.sciencephoto.com/media/962335/view

Lang, A., Habegger, M.L., & Motta, P. (2012). Shark Skin Drag Reduction. In Bhushan, B. (eds), Encyclopedia of Nanotechnology. Springer. https://doi.org/10.1007/978-90-481-9751-4_266

Lang, A. W., Motta, P., Hidalgo, P., & Westcott, M. (2008). Bristled shark skin: a microgeometry for boundary layer control? Bioinspiration & biomimetics, 3(4). DOI: 10.1088/1748-3182/3/4/046005 

Martin, S., & Bhushan, B. (2014). Fluid flow analysis of shark-inspired microstructure. Journal of Fluid Mechanics, 756, 5-29. DOI: 10.1017/jfm.2014.447 

Mo, Y., Xue, P., Yang, Q., Liu, H., Zhao, X., Wang, J., Jin, M., & Qi, Y. (2021). Composite Slow-Release Fouling Release Coating Inspired by Synergistic Anti-Fouling Effect of Scaly Fish. Polymers, 13(16). https://doi.org/10.3390/polym13162602

Rakers, S., Niklasson, L., Steinhagen, D., Kruse, C., Schauber, J., Sundell, K., & Paus, R. (2013). Antimicrobial Peptides (AMPs) from Fish Epidermis: Perspectives for Investigative Dermatology. Journal of Investigative Dermatology, 133(5). https://doi.org/10.1038/jid.2012.503

Scardino, A. J., & de Nys, R. (2011). Mini review: Biomimetic models and bioinspired surfaces for fouling control. Biofouling, 27(1). https://doi.org/10.1080/08927014.2010.536837

Schumacher, J. F., Carman, M. L., Estes, T. G., Feinberg, A. W., Wilson, L. H., Callow, M. E., Callow, J. A., Finlay, J. A., & Brennan, A. B. (2007). Engineered antifouling microtopographies – effect of feature size, geometry, and roughness on settlement of zoospores of the green alga Ulva. Biofouling, 23(1), 55–62. https://doi.org/10.1080/08927010601136957 

Soleimani, S., & Eckles, S. J. (2021). A review of drag reduction and heat transfer enhancement by riblet surfaces in closed and open channel flow. International Journal of Thermofluids, 9(1). https://doi.org/10.1016/j.ijft.2020.100053 

Xu, B., Wei, Q., Mettetal, M. R., Han, J., Rau, L., Tie, J., May, R. M., Pathe, E. T., Reddy, S. T., Sullivan, L., Parker, A. E., Maul, D. H., Brennan, A. B., & Mann, E. E. (2017). Surface micropattern reduces colonization and medical device-associated infections. Journal of Medical Microbiology, 66(11), 1692–1698. https://doi.org/10.1099/jmm.0.000600